Reduction of the general equation of the second degree to canonical form

SolitaryRoad.com

Website owner:  James Miller

[ Home ] [ Up ] [ Info ] [ Mail ]

REDUCTION OF THE GENERAL EQUATION OF THE SECOND DEGREE TO CANONICAL FORM

Any equation of the second degree

1) f(x, y, z) = ax² + by² + cz² + 2fyz + 2gxz + 2hxy + 2px + 2qy + 2rz + d = 0

can be reduced to one of 17 different canonical forms by a suitable translation and rotation. Each canonical form represents a quadric surface. Figure 1 shows a quadric surface (an ellipsoid) along with its canonical coordinate system x_c-y_c-z_c located at some point (x₀, y₀, z₀) in space (as referred to the X-Y-Z system). Reduction of a particular second degree equation to canonical form involves the following steps:

1] Determining the location (x₀, y₀, z₀) of the origin of the canonical system x_c-y_c-z_c of the surface.

2] Determining the orientation of the x_c-y_c-z_c system (as referred to the X-Y-Z system).

3] Determining the expression for our equation as expressed with respect to the x_c-y_c-z_c system by performing those substitutions associated with a translation of the X-Y-Z system to the point (x₀, y₀, z₀) and then a rotation to the orientation to the x_c-y_c-z_c system.

Determining the origin (x₀, y₀, z₀) of the canonical system. How is the point (x₀, y₀, z₀) found? If the quadratic surface has a center, point (x₀, y₀, z₀) corresponds to a center. A quadric surface may have a single center, a line of centers, or a plane of centers. If there is more than one center, translation to any center will do. Of the 17 quadric surfaces , 14 have centers. We compute the coordinates of a center using the equation

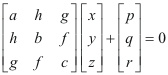
Three surfaces, the elliptic and hyperbolic paraboloids and the parabolic cylinder, do not have centers. In the case of these three, the point (x₀, y₀, z₀) corresponds to their vertices. The elliptic and hyperbolic paraboloids have a single vertex and the parabolic cylinder has a line of vertices. In the case of a line of vertices, translation to any vertex will do. These vertices must be found by some technique or procedure.

Determining the orientation of the canonical system. In the problem of translation to the canonical system origin we have noted that there may not be a single point that we must translate to but instead we may have a range of points that we can translate to (as in the case of a line of centers or a plane of centers). The same kind of situation exists in the problem of finding the orientation of the canonical coordinate system. In some cases there is a range of orientations that we can rotate to instead of just a single orientation. Consider the surface of revolution shown in Figure 2. Note that we can turn the canonical system x_c-y_c-z_c about the z_c axis through any angle from 0^o to 360^o and there is no change, one position is as good as another, the surface remains in canonical form. There is a range of acceptable directions for the x axis (and the y axis). This same thing will occur with any surface of revolution. With any surface of revolution there will exist a plane of acceptable directions for two of the axes corresponding to a rotation of the system about the third axis, the axis of symmetry. Now consider the case of a sphere located in space. In this case the canonical system can have any orientation. No matter how it is oriented the sphere is still in canonical form. Here we can choose any direction for the x axis and then rotate the system

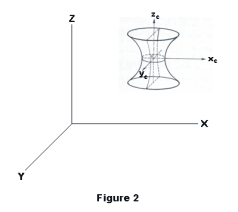
about the x axis to point the y axis in any direction we wish to give the system an orientation. Next question. How do we determine the directions of the x_c, y_c, and z_c axes of the canonical system? We compute a set of eigenvectors associated with the quadric surface. The directions of the eigenvectors give the directions of the canonical system axes. In the case of a surface of revolution where there is a plane of acceptable directions for two of the axes there will be a corresponding plane of eigenvectors. We arbitrarily pick one eigenvector in the plane for one axis and one perpendicular to it for the other axis (the eigenvectors radiate out in all directions from a point).