Website owner: James Miller
IMPROPER INTEGRALS
Def. Improper integral. The definite integral
is called an improper integral if
1] at least one of the limits of integration is infinite, or
2] the integrand f(x) has one or more points of discontinuity on the interval a
x
b.
Infinite limits of integration Integrals with one or more infinite limits are given meanings by the following definitions:
1] If f(x) is continuous on the interval a
x
h, we define
2] If f(x) is continuous on the interval h
x
b, we define
3] If f(x) is continuous on the interval h'
x
h, we define
If the limit (or limits) exist, an improper integral is said to be convergent. If a limit doesn’t exist the integral has no value and is said to be divergent.
Discontinuous integrand.
1] If f(x) is continuous on the interval a
x < b, but is discontinuous at x = b, we define
2] If f(x) is continuous on the interval a < x
b, but is discontinuous at x = a, we define
3] If f(x) is continuous for all values on the interval a
x
b except x = c, where a < c < b,
we define
Example 1. Evaluate the integral
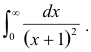
Solution. First we integrate from 0 to h thus obtaining a function of h. Then we examine the behavior of this function when h → ∞.
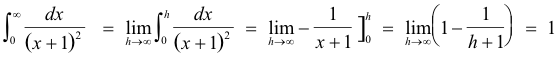
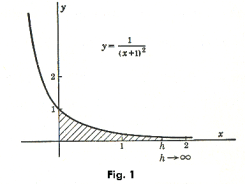
The graphical interpretation is shown in Fig. 1. The area under the curve
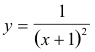
from x = 0 to x = h is
As the point h moves to the right, the area continually increases and approaches 1.
Example 2. Evaluate the integral
Solution. We will integrate from 2 to h and then examine the behavior of the resulting function of h as h → ∞.
The integral has no limit and is said to be divergent.
Theorem. Let
be an improper integral in which the function f(x) is discontinuous somewhere in the interval a
x
b . Let Φ(x) be the primitive of f(x) i.e. Sf(x)dx = Φ(x). If Φ(x) is continuous over the
interval a
x
b then integral 1) can be evaluated in the usual way of a regular, proper
integral i.e. without using ε. In other words, if Φ(x) is continuous on interval a
x
b
The proof for the case in which f(x) is continuous over the interval except for a vertical asymptote at x = k where a < k < b is as follows:
Because of the assumed continuity of Φ(x),
We then have
Proofs for the other cases are similar.
Example 3. Evaluate
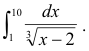
Solution. The function
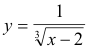
has a vertical asymptote at x = 2. However, the primitive
is a continuous function over the interval 1
x
10. We may then evaluate the integral in the
usual way:
Jesus Christ and His Teachings
Way of enlightenment, wisdom, and understanding
America, a corrupt, depraved, shameless country
On integrity and the lack of it
The test of a person's Christianity is what he is
Ninety five percent of the problems that most people have come from personal foolishness
Liberalism, socialism and the modern welfare state
The desire to harm, a motivation for conduct
On Self-sufficient Country Living, Homesteading
Topically Arranged Proverbs, Precepts, Quotations. Common Sayings. Poor Richard's Almanac.
Theory on the Formation of Character
People are like radio tuners --- they pick out and listen to one wavelength and ignore the rest
Cause of Character Traits --- According to Aristotle
We are what we eat --- living under the discipline of a diet
Avoiding problems and trouble in life
Role of habit in formation of character
Personal attributes of the true Christian
What determines a person's character?
Love of God and love of virtue are closely united
Intellectual disparities among people and the power in good habits
Tools of Satan. Tactics and Tricks used by the Devil.
The Natural Way -- The Unnatural Way
Wisdom, Reason and Virtue are closely related
Knowledge is one thing, wisdom is another
My views on Christianity in America
The most important thing in life is understanding
We are all examples --- for good or for bad
Television --- spiritual poison
The Prime Mover that decides "What We Are"
Where do our outlooks, attitudes and values come from?
Sin is serious business. The punishment for it is real. Hell is real.
Self-imposed discipline and regimentation
Achieving happiness in life --- a matter of the right strategies
Self-control, self-restraint, self-discipline basic to so much in life