Trigonometry: Definitions, formulas, identities, solution of triangles

SolitaryRoad.com

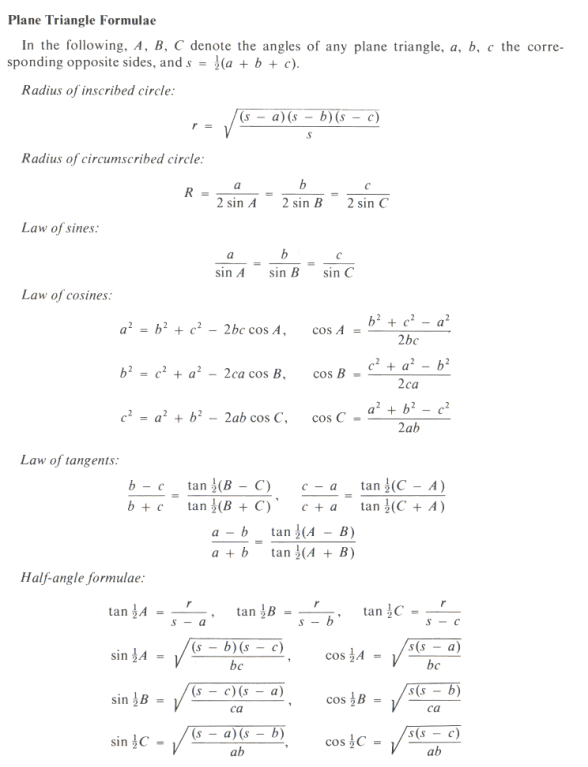
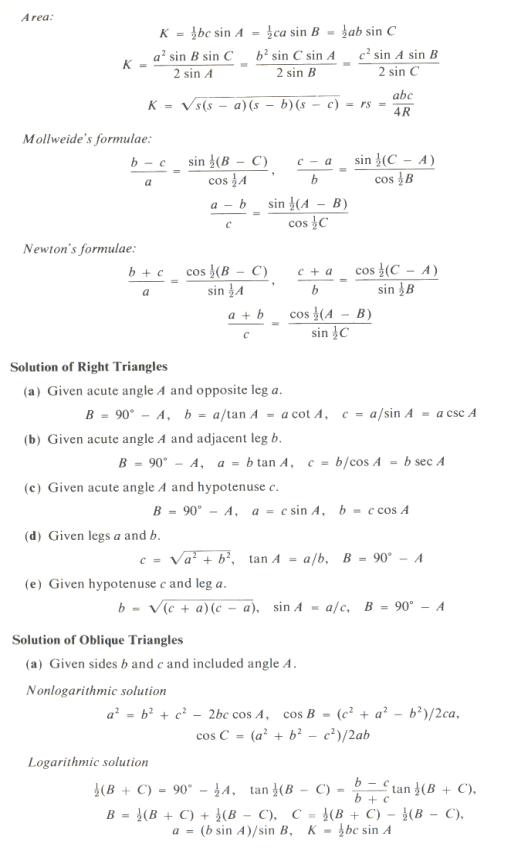
Website owner:  James Miller

[ Home ] [ Up ] [ Info ] [ Mail ]

TRIGONOMETRY: FORMULAS, IDENTITIES, SOLUTION OF TRIANGLES

Trigonometric functions of an acute angle. The basic definitions of the various trigonometric functions are given in terms of the acute angles of a right triangle. See Fig. 1. Shown is a right triangle in which C is the right angle, the side opposite being the hypotenuse c. In terms of this right triangle of Fig. 1 the definitions are as follows:

Trigonometric functions of complementary angles. The acute angles A and B of the right triangle ABC are complementary, that is A + B = 90^o. From Fig. 1 we have

These relations associate the functions in pairs — sine and cosine, tangent and cotangent, secant and cosecant — each function of a pair being called the cofunction of the other. Thus, any function of an acute angle is equal to the corresponding cofunction of the complementary angle.

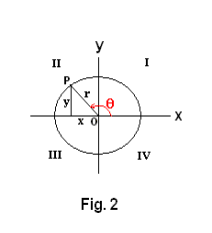
Trigonometric functions of a general angle. In the above definitions, the trigonometric functions are only defined for angles between 0^o and 90^o. For angles outside this range they are undefined. Using the above definition, the concepts make no sense for angles outside this range. In many of the applications of trigonometry we need to know the functions of angles greater than 90^o, as in the solution of oblique triangles. Angles up to 360^o are employed in navigation and surveying. The applications of trigonometric concepts are broad and diverse, cropping up in many fields, and both positive and negative angles of arbitrary size are typically encountered. For functions appearing in equations it is highly desirable that the function be defined for all values of the independent variable, not just some small range of values — and trigonometric functions frequently appear in equations. As a consequence the definition of the trigonometric functions has been broadened in such a way as to define meanings for the functions for all angles, not just the angles between 0^o and 90^o. The broadened definition utilizes the rectangular coordinate system shown in Fig. 2. Let P(x, y) be a point on a circle of radius r and let θ be the angle measured counterclockwise from the positive x axis to line OP. Then the trigonometric functions are defined as follows:

The coordinate system is divided into four quadrants — quadrants I, II, III, and IV --- as shown in the figure. The values of x are positive to the right of the origin and negative to the left of it, as usual, and the values of y are positive above the origin and negative below it. The signs of the functions change from quadrant to quadrant, depending on the signs of the x and y coordinates of point P. The angle θ can be either positive or negative, with the counterclockwise direction considered as positive, and is generally measured in either degrees or radians. Angles can be of any size — point P can make multiple trips around the origin.

It will be noted that for angles in the first quadrant the functions have the same values as in the previous definition.

Reciprocal relations. An immediate consequence of the definitions above are the following reciprocal relations:

sin θ = 1/csc θ tan θ = 1/cot θ sec θ =1/cosθ

cos θ = 1/sec θ cot θ = 1/tan θ csc θ = 1/sinθ