Surface integral representing a summation of flux passing through a closed surface

SolitaryRoad.com

Website owner:  James Miller

[ Home ] [ Up ] [ Info ] [ Mail ]

FLUX, VECTOR FLUX, SURFACE INTEGRAL REPRESENTING A SUMMATION OF FLUX PASSING THROUGH A CLOSED SURFACE

Def. Flux. The volume or mass of fluid or particles transferred across a given area perpendicular to the direction of flow in a given time.

There are many specific examples in science of the term flux. For electromagnetic radiation, it signifies the energy per unit time, or the power passing through a surface. For photons or particles, flux is the number per unit time passing through a surface.

Flux passing through a surface. If V describes a vector field, for example the velocity of an incompressible fluid, then the total flux through a surface S in the field is given by

∫∫_S V⋅dS

The vector V may refer to electric, magnetic, or gravitational force; heat or a fluid, etc. The surface integral may be converted to a volume integral by Gauss’s Theorem.

The International Dictionary of Applied Mathematics

Surface integral ∫∫_S A⋅dS representing a sum of the flux passing through a closed surface. Let A(x, y, z) be a vector point function representing a flux field defined over some region Q of space and S be some closed surface situated within Q. The surface integral

∫∫_S A⋅dS

can be given the physical interpretation

∫∫_S A⋅dS = ∑ all flux leaving surface S per unit time - ∑ all flux entering surface S per unit time

Here a summation is performed over the surface S in which all flux leaving the surface is given a plus sign and all flux entering is given a negative sign. If ∫∫_S V⋅dS has a positive value, it means more flux is leaving the surface than is entering it per unit time and flux must be being created within the surface. If ∫∫_S A⋅dS has a negative value, it means more flux is entering the surface than is leaving it per unit time and flux must be being destroyed within the surface. If ∫∫_S A⋅dS = 0, it means no flux is being created or destroyed within S.

Thus, if outward flow is regarded as positive and inward flow is regarded as negative, the integral ∫∫_S A⋅dS can be said to represent the net outward flow of flux from the surface per unit time.

Example. The flux could be that of a moving fluid such as water. The surface S could be a spherical cage constructed of wire mesh submerged in a stream or river. The water passing through the walls of the mesh cage would correspond to the flux A passing through the surface. In this case, the integral ∫∫_S A⋅dS would presumably have a value of 0 since, presumably, the amount of water entering the cage would be equal to the amount leaving the cage per unit time.

Examination of an example in which the flux is interpreted as the flow of a fluid.

Flux strength. The flux strength - i.e. the magnitude of A(x,y,z) - at a point can be viewed as a flow rate.

Def. Flow rate. The amount of fluid passing through a square unit of cross-sectional area perpendicular to the direction of flow per unit of time. Example: Three cubic feet of water pass through a one square foot cross-sectional area perpendicular to the direction of flow per second. The flow rate is three cu. ft/sec/sq. ft.

The product of flow rate times a cross-sectional area perpendicular to the direction of flow gives the amount of fluid passing through the cross-sectional area per unit time.

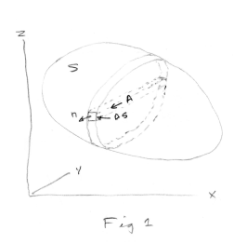
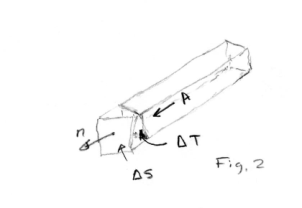
Consider the flux A at some small surface element ΔS on the surface of S. A represents the amount of fluid that passes through one square unit of cross-section perpendicular to A in one second. See Fig. 1. The vector A can be written as A = |A|a where a is a unit vector in the direction of A and |A| is the magnitude of A. The amount of fluid passing through surface element ΔS per second is given by |A| ΔT where ΔT is the area of the projection of surface element ΔS on a plane perpendicular to A. See figures 2 and 3. ΔT is the area of the region ABCD shown in Fig. 3. ABCD represents the projection of the surface element ΔS on a plane through point A perpendicular to vector A. We will denote the area of element ΔS by the same name, ΔS. ΔT is related to the area ΔS by

ΔT = n∙a ΔS

where n is the unit normal to ΔS. Proof.

In differential form this can be written

dT = n∙a dS .

The sum of all the flux passing through the wall of the closed surface S per second is then given by

∫∫_S |A| dT = ∫∫_S |A| n⋅a dS = ∫∫_S A⋅n dS

Regarding dS as a vector normal to the surface, we can write

∫∫_S A⋅n dS = ∫∫_S A⋅dS

Thus the sum of all the flux passing through the wall of the closed surface S per second is then given by ∫∫_S A⋅dS .