Affine transformations

SolitaryRoad.com

Website owner:  James Miller

[ Home ] [ Up ] [ Info ] [ Mail ]

Transformations of the plane.

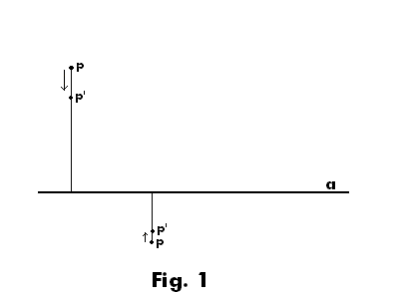
Uniform “contraction” of the plane toward a line. One of the simplest transformations of the plane is what we can describe as a uniform “contraction” of the plane toward a line. We describe now exactly what we mean by this. Suppose we are given a line a in the plane and a positive coefficient k. Assume, for example, that k = 3/4. By a uniform contraction, with coefficient k = 3/4, of the plane toward line a we mean that every point P of the plane is sent into point P' in such a way that P' lies on the same side of the plane as P, lies on the perpendicular from P to a, and is k = 3/4 of the distance from P to a. See Fig. 1. In the example we have just used k is less than one, and we have a proper contraction of the plane toward the line. If k is greater than one we would have an expansion of the plane from the line, instead of a contraction. We will, however, use the word “contraction”, putting it in quotation marks, to represent both cases.

Properties of uniform “contraction” transformations.

Under a uniform “contraction” of a plane toward a line:

1) Any straight line of the plane is transformed into a straight line.

2) Parallel lines remain parallel.

3) If a point divides a segment in a given ratio, the image of the point divides the image of the segment in the same ratio.

“Contractions” of the plane to a line are a special case of more general, so-called affine transformations of the plane.

Affine transformation of the plane. The linear transformation

x' = a₁ x + b₁y + c₁

y' = a₂ x + b₂y + c₂

is an affine transformation of the plane provided the matrix

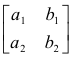
is non-singular.

Affine transformation of three-dimensional space. The linear transformation

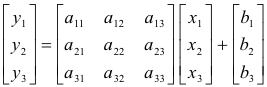
is an affine transformation of space provided the matrix

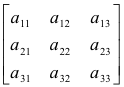
is non-singular.

Generalized Cartesian coordinate systems. We call a pair of vectors e₁, e₂, starting from a common origin O and not lying on the same line, a “coordinate frame” of the plane. The coordinates of a point P relative to this frame Oe₁e₂ are given by the numbers x, y such that in order to reach the point P from the origin O one lays off from the point O x-times the vector e₁ and then y-times the vector e₂. Such a coordinate frame represents a generalization of the usual Cartesian rectangular coordinate system. The usual Cartesian system is a special case of this generalized system in which the coordinate vectors e₁, e₂ are mutually perpendicular and of unit

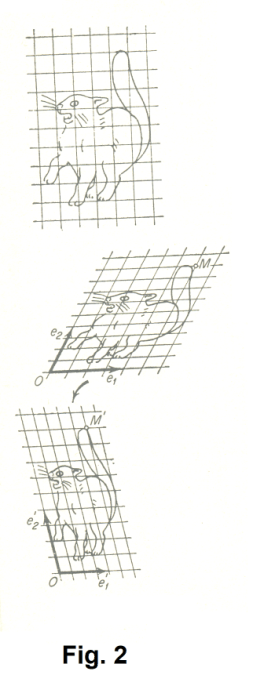
length. A similar generalized coordinate system can be introduced in three-dimensional space using three vectors e₁, e₂, e₃ emanating from a common point O.

In an affine transformation of the plane a given coordinate frame Oe₁e₂ is transformed into a certain other coordinate frame Oe₁'e₂' (generally speaking, with another “metric” i.e. with different lengths for the vectors e₁' and e₂' and a different angle between them) and an arbitrary point M is sent into the point M' having the same coordinates relative to the new frame as M had relative to the old. See Fig. 2. In such a transformation a given net of equal parallelograms is transformed into another arbitrary net of equal parallelograms.

In an affine transformation of space a given coordinate frame Oe₁e₂e₃ is transformed into a certain other coordinate frame Oe₁'e₂' e₃'(generally speaking, with another “metric” i.e. with different lengths for the vectors e₁'_,e₂' and e₃' and different angles between them) and an arbitrary point M is sent into the point M' having the same coordinates relative to the new frame as M had relative to the old.

Mathematics, Its Content, Methods and Meaning. Vol. 1, p. 231- 232

Properties of affine transformations.

- straight lines are mapped into straight lines, parallel lines are mapped into parallel lines, and if a point divides a segment in a given ratio, the image of the point divides the image of the segment in the same ratio.

Theorem. Any affine transformation of the plane can be obtained by performing a certain rigid motion of the plane onto itself, and then, in general, two uniform “contractions” with different coefficients k₁ and k₂ toward two mutually perpendicular lines. Similarly, any affine transformation of three-dimensional space can be obtained by performing a certain rigid motion of the space onto itself, and then, three uniform “contractions” toward three mutually perpendicular planes with certain coefficients k₁, k₂, k₃.

References.

Mathematics, Its Content, Methods and Meaning. Vol. I