Website owner: James Miller
Linear transformation Y=AX viewed as a product of rotations and elongations /contractions in mutually orthogonal directions
Consider the case of a two dimensional transformation Y = AX and consider what it transforms a unit circle centered at the origin into. It transforms the circle into an eclipse where the semi-major and semi-minor axes of the ellipse correspond to the directions of the mutually perpendicular elongations / contractions. Also, the semi-major and semi-minor axes represent the images of a certain two diameters of the unit circle which also happen to be mutually perpendicular. The linear transformation is equivalent to a rotation of the x-y system to bring the x axis into coincidence with diameter AB, two elongations / compressions in the x and y directions (which stretch and compress the circle into the shape of the ellipse) and a final rotation that carries the ellipse into its final position.
Unit vectors in the direction of
and
are given by the normalized eigenvectors of the
matrix (AAT) -1 . See figure. Unit vectors in the direction of
and
are given by the
normalized eigenvectors of the matrix (AT A) -1 . If B is a matrix whose columns consist of the
normalized eigenvectors of (AAT) -1 and C is a matrix whose columns consist of the normalized
eigenvectors of (AT A) -1 then A can be written as the product:
A = BDC -1
where D is a diagonal matrix. C effects the first rotation, D effects the two perpendicular elongations / compressions, and B effects the final rotation.
______________________________________________________________________
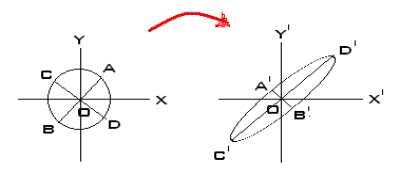
Re-examination and re-working of the same problem. The following represents a re-examination and reworking of the same problem done in June 84.
Consider the following point transformation in the plane: Let a unit circle centered at the origin
1)
or, in matrix form,
be transformed by the linear point transformation
where A is a non-singular 2x2 matrix.
The equation of the transformed circle will be
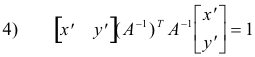
or, equivalently,
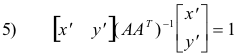
and will be an ellipse. See figure.
Let λ1 and λ2 be the eigenvalues of matrix (AAT)-1 . Let B be a matrix whose columns consist of
the corresponding normalized eigenvectors of the matrix (AAT)-1 . The columns of B will then
consist of elementary unit vectors along the axes
and
.
The lengths of the semi-axes
and
are given by the quantities
and
respectively.
Let a matrix C be given by
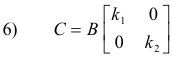
The columns of C consist of the vectors
and
.
Let a matrix D be given by
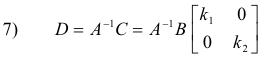
The columns of D consist of the vectors
and
.
If we rearrange 7) above we arrive at the following factorization of the matrix A:
Since matrix D is orthogonal 8) may be written as
which is our final result.
In the linear transformation
matrices B and DT are both orthogonal matrices effecting rotations of the coordinate system and
matrix
effects stretching / compressing in two mutually perpendicular directions. Matrix
DT takes a point expressed with respect to the x-y coordinate system and expresses it with respect
to the B-O-D coordinate system, matrix
effects stretching /shrinking in the directions of the
and
axes, and matrix B takes a point expressed in the B-O-D coordinate system and
expresses it in the final x’-y’ coordinate system.
General case when A is an nxn matrix. We have developed the formula for the factorization of matrix A for the case of two dimensional space. The case for n-dimensional space is a direct extension of the above reasoning.
Let a unit sphere in n-dimensional space
= 1
or, equivalently,
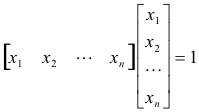
be transformed by the linear point transformation
X' = AX
The equation of the transformed sphere will be
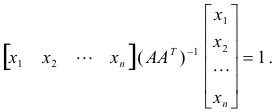
Let λ1, λ2, .... , λn be the n eigenvectors of the matrix (AAT) -1 and B be a matrix whose columns consist of the corresponding normalized eigenvectors of (AAT) -1 .
Let
Let
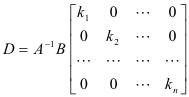
Then
which is our desired factorization.
Matrices B and DT are both orthogonal matrices effecting “rotations” in n-dimensional space and matrix
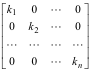
effects stretching / compression in n mutually orthogonal directions in n-dimensional space.
Jesus Christ and His Teachings
Way of enlightenment, wisdom, and understanding
America, a corrupt, depraved, shameless country
On integrity and the lack of it
The test of a person's Christianity is what he is
Ninety five percent of the problems that most people have come from personal foolishness
Liberalism, socialism and the modern welfare state
The desire to harm, a motivation for conduct
On Self-sufficient Country Living, Homesteading
Topically Arranged Proverbs, Precepts, Quotations. Common Sayings. Poor Richard's Almanac.
Theory on the Formation of Character
People are like radio tuners --- they pick out and listen to one wavelength and ignore the rest
Cause of Character Traits --- According to Aristotle
We are what we eat --- living under the discipline of a diet
Avoiding problems and trouble in life
Role of habit in formation of character
Personal attributes of the true Christian
What determines a person's character?
Love of God and love of virtue are closely united
Intellectual disparities among people and the power in good habits
Tools of Satan. Tactics and Tricks used by the Devil.
The Natural Way -- The Unnatural Way
Wisdom, Reason and Virtue are closely related
Knowledge is one thing, wisdom is another
My views on Christianity in America
The most important thing in life is understanding
We are all examples --- for good or for bad
Television --- spiritual poison
The Prime Mover that decides "What We Are"
Where do our outlooks, attitudes and values come from?
Sin is serious business. The punishment for it is real. Hell is real.
Self-imposed discipline and regimentation
Achieving happiness in life --- a matter of the right strategies
Self-control, self-restraint, self-discipline basic to so much in life