Website owner:  James Miller

Prove. The equivalent capacitance C_E of n capacitors C₁, C₂, ... , C_n connected in parallel is

1)        C_n = C₁ + C₂ + ... + C_n

where C₁, C₂, ... , C_n are the capacitances of the capacitors.

Proof. We will prove it for three capacitors C₁, C₂, and C₃.

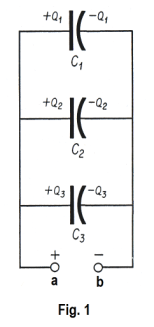
Let a potential difference V_ab be applied across the terminals a and b of the parallel network shown in Fig. 1. The potential difference across each of the capacitors is V_ab, however the charges on the different capacitors is different. The charges on the capacitors are

2)        Q₁ = C₁V_ab                Q₂ = C₂V_ab                Q₃ = C₃V_ab

The total charge Q on the network is

3)        Q = Q₁ + Q₂ + Q₃

The equivalent capacitance C_E is that of a single capacitor which would acquire the same total charge Q with the same potential difference. Thus                                                                      

4)        Q = C_EV_ab

Substituting 2) and 4) into 3) gives

5)        C_EV_ab = C₁V_ab + C₂V_ab + C₃V_ab

or

6)        C_E = C₁ + C₂ + C₃

We note that the energy of the single equivalent capacitor is equal to the sum of the energies of capacitors C₁, C₂, and C₃. The energy of the equivalent is ½ QV_ab and the sum of the energies of

capacitors C₁, C₂, and C₃ is ½ Q₁V_ab + ½ Q₂V_ab + ½ Q₃V_ab = ½ QV_ab .